
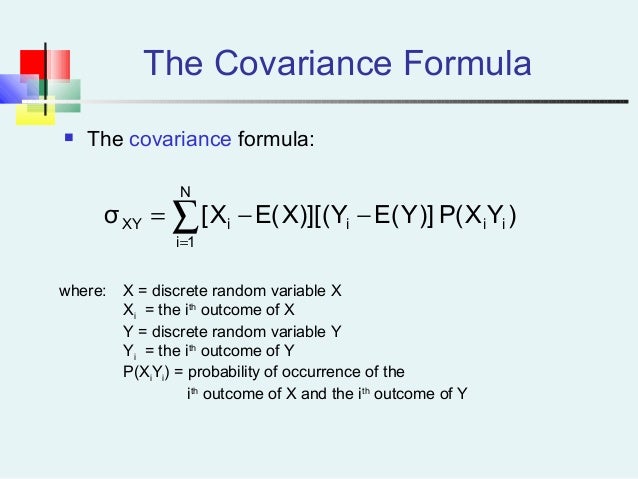
That is why I mentioned HHHHHH and HHHHHT and would like to see Don solve it his way. One can see why HH is longer than HT because a T forces the system to go all the way back to 0 I could show a diagram of the transition matrix and some will get it. There may not be enough time to explain so all gets it either.
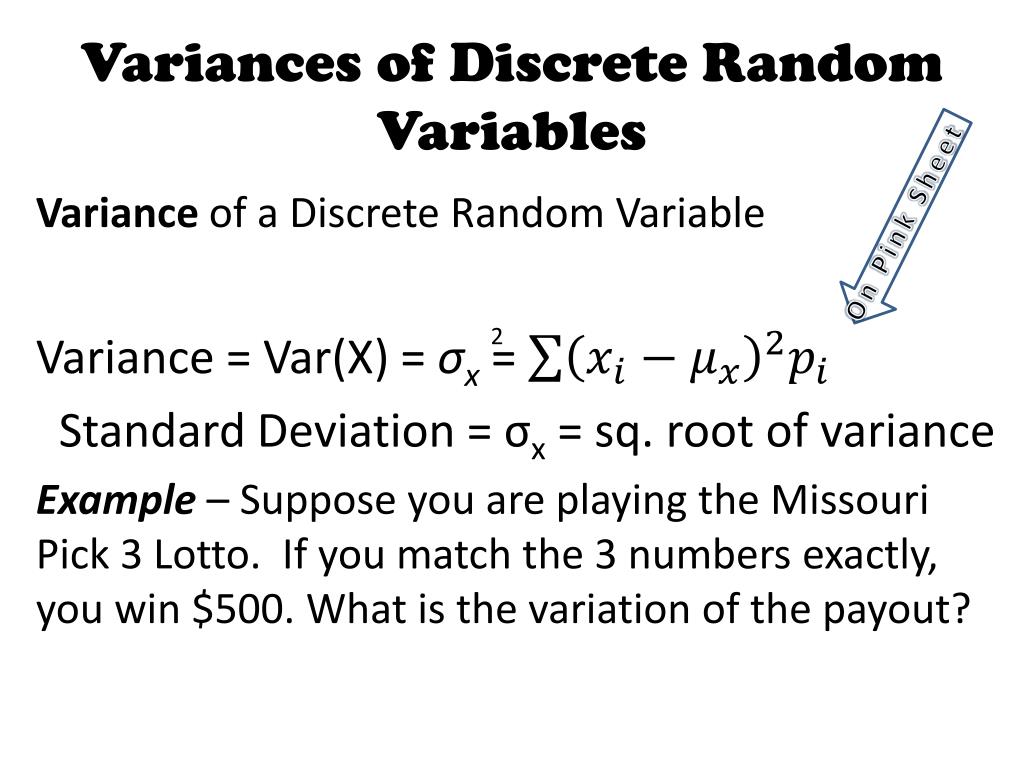
I also realize, the vast majority is lost with how I solved the HT or HH question. It is basic math and gives lots of info, more than just xĪnd, to me, they are fun to do, sometimes My perfered method for these type Qs is to use a Markov chain solution. just what I use as a different way to get an answer. I studied your solution but did not acknowledge it because I thought it veered off the point. In this case the expected number of flips is e + 2 with a probability of 1/4Į = (1/2)(e + 1) + (1/4)(e + 2) + (1/4)(2) We are back to square one and cannot complete our goal in fewer than two additional flips. We have attained our goal in two flips with a probability of 1/4.Ģbb. First flip is H: we are half way there waiting for the next flip.Ģba. First flip T: this is useless, so e is increased by one.Ģb. In this case we expect to attain our goal in an average of 4 flips.Īdding up these three contingencies weighted by their probabilities:Ģa. That would be a T and the average number of flips to get a T is 2. We are at HH and can attain our goal in one more flip. The next flip is T and we have attained our goal in two flips with a probability 1/4.ġbb. First flip T: this is useless, so e is increased by one.ġba. Let e be the expected (average) number of flips to achieve the goal.ġa. This game was published as Problem 95 by Walter Penney in the Journal of Recreational Mathematics (1969)." A collection of active words is given, and a coin is flipped to see which word from the list is the first to appear in the list of results.
"Flipping a coin results in either an “H” or a “T” a sequence of consecutive flips is thereby represented by a word. That is a game that just is not that intuitive at first sight (Penney Ante game) This is way different from a 'race' between 2 patterns or more The worksheet I linked to has a sheet that shows this as well Xxxxx H <- matches the basic pattern starting at 6th flip Xxxx HH <- matches the basic pattern starting at 5th flip Xxx HHT <- not a match with the basic pattern starting at 4th flip Xx HHTT<- not a match with the basic pattern starting at 3rd flip X HHTTH <- not a match with the basic pattern starting at 2nd flip The overlap concept (in patterns) for many is difficult to grasp Here is a link to a spreadsheet that shows average number of trials for any value of pįor a 2 to 6 word sequence (pattern) I think I got all the errors out of it. This was (first ?) talked about by a famous math guy (John Conway) but can also be seen in a simple Markov chain transition matrix. There is overlap in the pattern (sequence) and we can easily calculate that event (those events) If p=1/2 for an H we can easily say 1/p = the average number of flips to get an H = 2 I see the patterns H and HH as way different. There is a general rule that the average number of times an event with a probability of p will come up is 1/p,the general rule has to do with independent events.


 0 kommentar(er)
0 kommentar(er)
